ZYNQ开发实例之布局约束
本文共 739 字，大约阅读时间需要 2 分钟。
分享一例位置约束实例
如下图所示，出现这个警告的意思是在一个bank中时钟管理器不够用需要到其他bank中找一个，但这不是最优的解决方案，如果要这么处理就需要在约束文件中进行约束
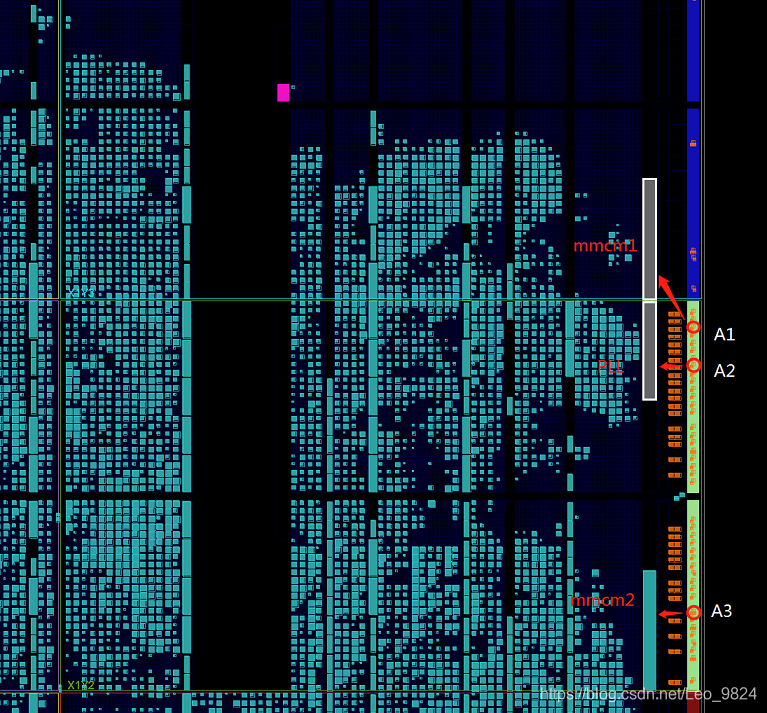
先分配一下IO对应的时钟管理器。A1分配mmcm1，A2分配PLL，A3分配mmcm2，如下图：
可以在属性中获取时钟管理器的坐标：
mmcm1的坐标是MMCME2_ADV_X1Y3 pll的坐标是PLLE2_ADV_X1Y2 mmcm2的坐标是MMCME2_ADV_X1Y2可以在Schematic中找到时钟管理器的网络路径：
鼠标选中目标器件，在tcl中输入get_selected_objects命令就会打印出目标路径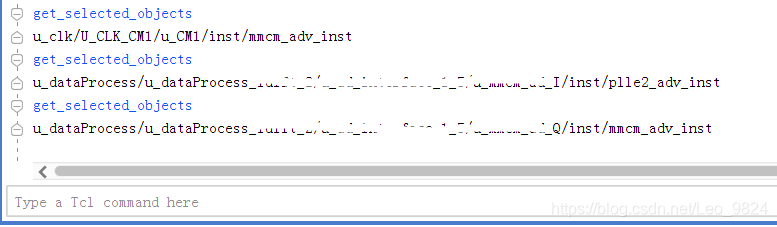
约束： 消除警告
set_property CLOCK_DEDICATED_ROUTE BACKBONE [get_nets clk_9516]位置约束
set_property LOC MMCME2_ADV_X1Y3 [get_cells u_clk/U_CLK_CM1/u_CM1/inst/mmcm_adv_inst]位置约束
set_property LOC PLLE2_ADV_X1Y2 [get_cells u_dataProcess/u_dataProcess_1dfft_2/u_ad_interface_1_5/u_mmcm_ad_I/inst/plle2_adv_inst]位置约束
set_property LOC MMCME2_ADV_X1Y2 [get_cells u_dataProcess/u_dataProcess_1dfft_2/u_ad_interface_1_5/u_mmcm_ad_Q/inst/mmcm_adv_inst]转载地址：http://jzfiz.baihongyu.com/
你可能感兴趣的文章
mt_rand
查看>>
mysql -存储过程
查看>>
mysql /*! 50100 ... */ 条件编译
查看>>
mudbox卸载/完美解决安装失败/如何彻底卸载清除干净mudbox各种残留注册表和文件的方法...
查看>>
mysql 1264_关于mysql 出现 1264 Out of range value for column 错误的解决办法
查看>>
mysql 1593_Linux高可用(HA)之MySQL主从复制中出现1593错误码的低级错误
查看>>
mysql 5.6 修改端口_mysql5.6.24怎么修改端口号
查看>>
MySQL 8.0 恢复孤立文件每表ibd文件
查看>>
MySQL 8.0开始Group by不再排序
查看>>
mysql ansi nulls_SET ANSI_NULLS ON SET QUOTED_IDENTIFIER ON 什么意思
查看>>
multi swiper bug solution
查看>>
MySQL Binlog 日志监听与 Spring 集成实战
查看>>
MySQL binlog三种模式
查看>>
multi-angle cosine and sines
查看>>
Mysql Can't connect to MySQL server
查看>>
mysql case when 乱码_Mysql CASE WHEN 用法
查看>>
Multicast1
查看>>
mysql client library_MySQL数据库之zabbix3.x安装出现“configure: error: Not found mysqlclient library”的解决办法...
查看>>
MySQL Cluster 7.0.36 发布
查看>>
Multimodal Unsupervised Image-to-Image Translation多通道无监督图像翻译
查看>>